E. Level Network Adjustment

1. Misclosure

a. Determining

A closed level network is either a loop or link which begins and ends on a known elevation, Figure E-1.

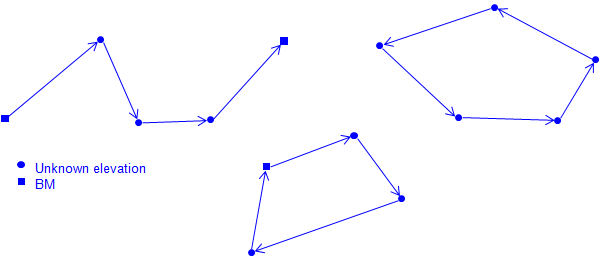Figure E-1

In the process of running the network, new elevations are measured including that of the known ending elevation. The difference between the known and measured ending elevation allows us to determine the amount of error, or misclosure, in the network:

Misclosure should be the result of accumulated random errors. Systematic errors and mistakes must be eliminated first in order to judge quality of the newly created elevations. Random errors, while they can't be entirely eliminated, can be minimized by knowledgeable personnel using the appropriate equipment and procedures under the best conditions.

b. Allowable

How much misclosure is acceptable? It depends on the purpose of the network. The allowable misclosure should be consistent with how random errors accumulate. Differential leveling consist of a series of instrument setups at which two rod readings are made. This is repeated throughout the entire network. If we assume that each time the process is performed we expect a certain error behavior then we can consider the total misclosure to behave like an Error of a Series.

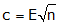	Equation E-2
c: total error E: expected error for a condition n: number of times the condition occurs

(1) Formal standards

Table E-1 is taken from Section 3.5 Geodetic Leveling of Standards and Specifications for Geodetic Control Networks, FGCC, 1984.

Table E-1

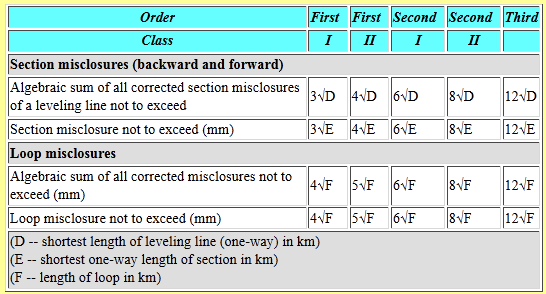 The Loop misclosure not to exceed (mm) row identifies the network misclosure using a form of the Error of a Series equation. The allowable misclosure is based on the loop length (that is, the total of all the BS and FS distances) instead of instrument set ups. Consider the greater difficult reading a level rod at twice the distance - not only are rod divisions smaller but other environmental factors add to the difficulty as well. Assuming error accumulates as a function of distance is reasonable.

Example

The distance around a level network is approximately 1800 ft. What is the allowable misclosure, in feet, if the network is to meet Second Order Class II standards?

Convert the distance to km

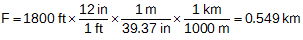
Compute the misclosure, m, and convert to feet

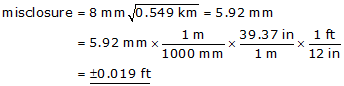
(2) Informal standards

Informal standards can also be based on the Error of a Series. However, the error accumulation could be presumed based on distance surveyed or number of instrument setups.

Example

A survey crew ran a level network consisting of seven set ups. If they have an expected error of ±0.005 ft for each set up, what is their expected network misclosure?

Using Equation E-2:

Whether using formal or informal standards, remember that the final results are affected by errors from the different sources. Controlling those errors is paramount to meeting misclosure standards by design, not by mistake.