2. Types of Horizontal Angles

a. Interior/Exterior

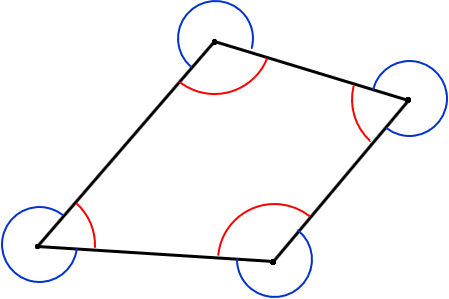
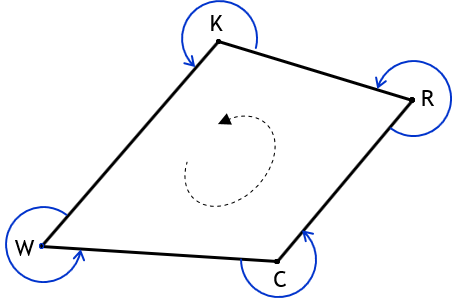
On a closed non-crossing polygon, Figure G-5, horizontal angles can be either interior (red) or exterior (blue).

The geometric condition for a non-crossing polygon is Equation (G-1).

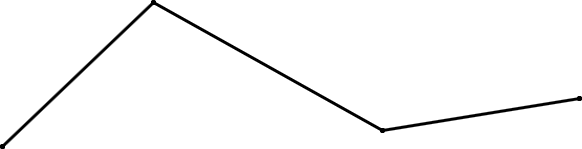
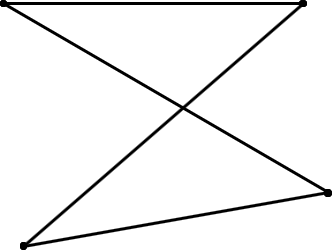
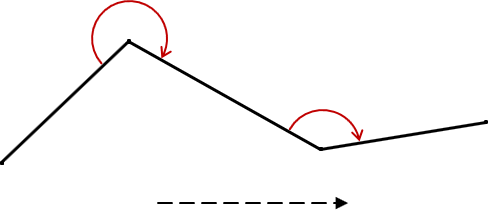
Interior and exterior don’t make sense if the polygon is open, Figure G-6(a), or if the polygon crosses itself, Figure G-6(b).

In the latter case, the polygon turns itself inside-out and Equation (E-1) does not apply. Open and crossing polygons need some other way to express an angle.

b. Right/Left

Right or left is the rotational direction from the BS point to the FS point. Consider standing on the At point, looking at the BS point: an angle to the right means you physically turn your body to the right to see the FS point. An angle right is clockwise, left counter-clockwise, Figure G-7.

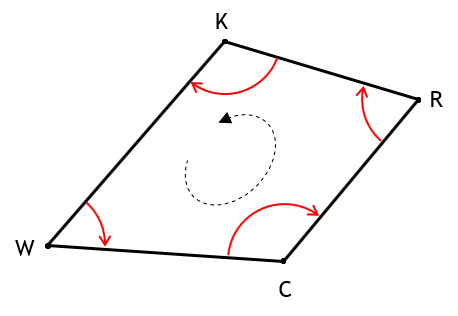
Figure G-8(a) shows a closed non-crossing polygon with interior angles to the right; Figure G-8(b) to the left.

The dashed lines in Figure G-8 show the angle measurement sequence. In Figure G-8(a), interior angles to the right measurement progresses counter-clockwise around the polygon.

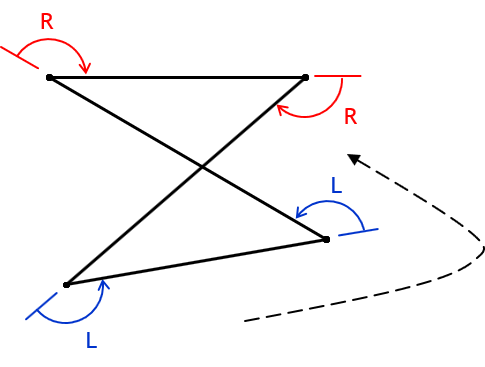
We can also have exterior angles to the right, Figure G-9(a), and to the left, Figure G-9(b).

Right or left help with angle definitions on the open and crossing polygons. In Figure G-10, progressing in the direction of the dashed line, the open polygon has angles to the right.

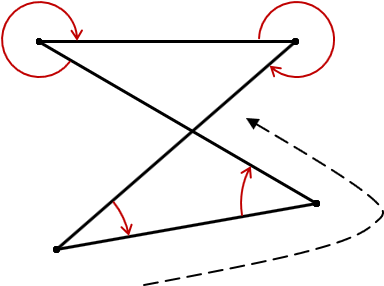
Figure G-11 shows a crossing polygon using angles left progressing in the direction of the dashed line.

Note how the angles change from “interior” to “exterior” although there is no consistent polygon interior.

c. Deflection Angles

A deflection angle is how much the next line deflects from an extension of the previous line. It consists of two parts: (1) magnitude and (2) direction. For example, in Figure G-12:

Line BC deflects from line AB 45° to the right, written as 45°R.

Line CD deflects from line BC 30°L.

A deflection angle ranges from 0° (no deflection) to 180° (going back along the preceding line).

The mathematical angle condition for a closed non-crossing polygon, Figure G-13, is:

Right deflection angles are positive (+), left are negative (-).

Equation (G-2) is also the mathematical condition for a closed polygon which crosses itself an even number of times. For an odd number of crossings, Figure G-14, the mathematical condition is:

How does reversing travel direction around a polygon change the deflection angles? Figure G-15 shows that the deflection angle value is the same, but its direction is reversed.