3. Angular Misclosure Distribution
Normally, the first step in traverse computations is distribution of the angular misclosure in order to meet the angular condition. This is referred to as angle adjustment. If performing a least squares traverse adjustment, angles are not adjusted since the angular misclosure is part of the overall adjustment.
Because angular misclosure is due to random errors, a distribution method which approximates their behavior would be the best to use. There are a few simplified methods which can be applied, each having advantages and disadvantages, Table B-2:
| Table B-2 | |||
| Method | Process | Advantage | Disadvantage |
|
Don’t distribute |
- - |
Simple; Can be replicated. |
Unless using a least squares adjustment, this method does not try to reconcile error accumulation. |
|
Arbitrary |
Put all error into random angle. |
None. |
Doesn't model errors well; Difficult to replicate. |
|
Equal distribution |
Apply equal correction to each angle. |
Simple; Can be replicated. |
Assumes error is same in each angle; Can imply incorrect angle accuracy. |
|
Judgment |
Apply different corrections to each angle depending on measurement repetition and conditions. |
Relatively simple; Better error modeling. |
Requires direct knowledge of measurement conditions. |
Why isn't Least Squares listed as a distribution method? Because angles aren't adjusted separately when a traverse is adjusted by least squares: angles and distances are adjusted simultaneously.
One thing we do not base corrections on is angle size. An angle is the difference between a total station’s initial and final readings; size of the angle has no impact on its error.
Example
Given the following traverse and measured angles:
 |
|
||||||||||||||
| Figure B-6 Example Angle Adjustment |
|||||||||||||||
The angle condition from Equation B-1 is
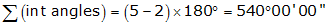

Since the angle total is short of the angle condition, corrections must be added.
Using an Equal Distribution

Apply correction:
|
Point |
Angle |
corr’n |
Corr’d Angle |
|
A |
125°30'20" |
+0°00'02.6" |
125°30'22.6" |
|
B |
88°21'31" |
+0°00'02.6" |
88°21'33.6" |
|
C |
112°38'35" |
+0°00'02.6" |
112°38'37.6" |
|
D |
104°21'40" |
+0°00'02.6" |
104°21'42.6" |
|
E |
109°07'41" |
+0°00'02.6" |
109°07'43.6" |
|
sums: |
539°59'47" |
+0°00'13.0" |
540°00'00.0" |
|
check |
check |
While the corrected angles total the angle condition, each is shown to 0.1" which implies a higher accuracy than shown in the original angles. But rounding each to the nearest second raises the total to 540°00'05".
Using Judgment
In this case, we take into account measurement conditions.
Consider point E, which has short sights on both sides. Shorter sights generally have higher pointing errors (given same target sizes).
Also, the field crew reported larger repeated direct and reverse angle spreads at point C.
The equal distribution’s correction was +0°00'02.6" per angle. We can drop that to +0°00'02" per angle for our three strongest angles (+0°00'06" total) and divide the remaining 0°00'07" among the two weaker ones.
|
Point |
Angle |
corr’n |
Corr’d Angle |
|
A |
125°30'20" |
+0°00'02" |
125°30'22" |
|
B |
88°21'31" |
+0°00'02" |
88°21'33" |
|
C |
112°38'35" |
+0°00'04" |
112°38'39" |
|
D |
104°21'40" |
+0°00'02" |
104°21'42" |
|
E |
109°07'41" |
+0°00'03" |
109°07'44" |
|
sums: |
539°59'47" |
+0°00'13" |
540°00'00" |
|
|
|
check |
check |
This takes into account individual conditions. The resulting angles do not imply accuracy beyond the original measurements.
Equal Distribution or Judgement?
Although an equal distribution can result in decimals beyond original accuracy, that's really not an issue. Why? Because balanced angles are an intermediate calculation so carrying additional digits reduces cumulative rounding error. In most simple traverse cases, an equal distribution works fine.
Distribution based on judgement should be used where there is personal knowledge of measurement conditions and angle certainty.