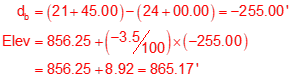Solutions: Basic Components

Problem (1)

What is the distance in feet from station 23+56.38 to 30+06.85?

Dist = (30+06.85) - (23+56.38) =   650.47'  

Problem (2)

A 1750.00 ft long alignment starts at 12+45.64. What is the end station of the alignment?

Sta = (12+45.64) + (17+50.00) =   29+95.64 

Problem (3)

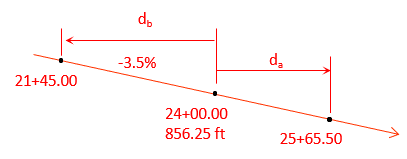
A -3.50% grade begins at station 21+45.00 and at station 24+00.00 passes through an elevation of 856.25. The grade line terminates at station 25+65.50.

Problem (4)

Compute the grade between the following points

	From: sta @ elev	To: sta @ elev
Part (a)	12+50.00 @ 968.23'	16+75.00 @ 975.23'
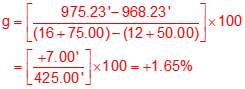Part (b)	37+76.35 @ 1048.64'	39+18.42 @ 1042.60

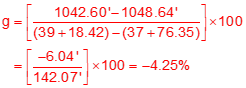 Problem (5)

A short straight alignment begins at station 23+50.00, whose coordinates are 8000.00' N, 3000.00' E, and runs S38°20'45"W 735.30' to its end. A survey crew located an existing power pole and reported its position as 76.44 ft L @ 28+02.35.  What are the coordinates of the power pole?

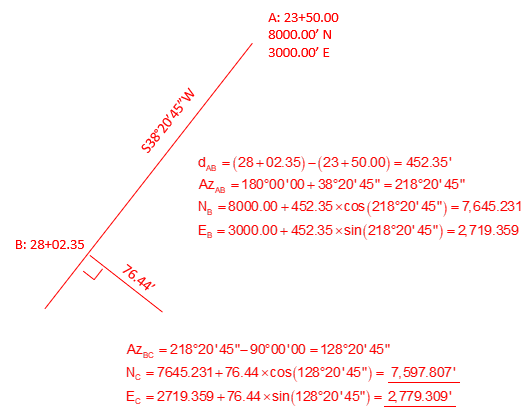
Problem (6)

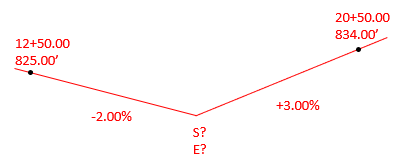
A -2.00% grade leaves from station 12+50.00 at an elevation of 825.00. It intersects a +3.00% grade line which ends at station 20+50.00 and elevation 834.00. What are the station and elevation at the intersection of the two grade lines?

Sketch:

Write the grade equation for each line. Rearrange in terms of E.

Set the equations equal, solve for E.
Then substitute back into one of the grade equations to get S.

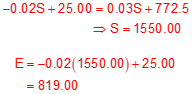
Answer: Station = 15+50.00, Eleation = 819.00'